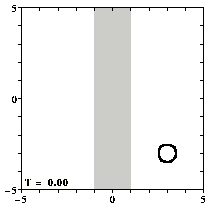
Contours of relative vorticity of the initial situation.
The location of the ridge is shown by the shaded region.
(You can click on the pictures for a larger version.)

A monopolar vortex, such as the Bessel monopole, will move in the presence of a background rotation, due to the Coriolis force, as explained on the page about a Bessel monopole on a beta-plane. The rotation effects are described in the model with the Coriolis parameter f=f0+beta*y. The value of f0 does not matter for that simulation since there is no bottom topography example. If there is a bottom topography, then things can be quite different.
In the simulations presented here the monopole encounters a north-south oriented ridge, as it travels to the north-west due to the beta-effect.

Contours of relative vorticity of the initial situation.
The location of the ridge is shown by the shaded region.
(You can click on the pictures for a larger version.)
For all graphs: positive contours (0.1 to 2.0 at an interval of 0.1) are drawn solid, negative contours (-0.1 to -2.0 at an interval of -0.1) are drawn dashed, and the zero contour is dotted. Since the maximum of vorticity at the centre of the monopole is much larger than 2.0, the vortex looks like a "hole".
Note that the domain measures 20 by 20 length units -- the graphs show only the central part of this domain.
Turning on the time evolution means that the monopole moves to the north-west, due to the beta-effect, and it encounters the ridge:
The monopole then starts climbing the ridge. But the effect of the topography is quite strong and the monopole moves along the ascending (east) side of the ridge to the north, while it is being deformed heavily:
Eventually there is again a clear vortex on the west side of the top of the ridge and after this vortex has descended the ridge it continues it beta-induced motion to the north-west:
This MPEG movie (0.5 Mb; 101 frames) featuring the evolution from T=0 until T=50, shows far more clearly the effect of the ridge on the monopole.
There is also a colour version of the MPEG movie, which is nice to look at but shows the Rossby waves less clear.
As the monopole moves, it leaves behind vorticity in the form of so-called Rossby waves, and the monopole interacts with this vorticity again, leading to a non-straight motion to the north-west. When the monopole has reached the ridge, it starts moving more to the north than it would have done without the ridge (cf. the page on the Bessel monopole on a beta-plane) and at the same time the vortex becomes weaker (as explained on the page about the effect of a bottom topography). For this simulation:
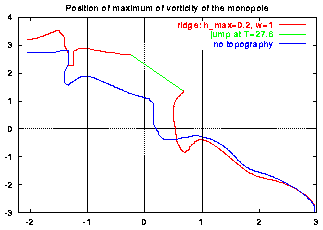
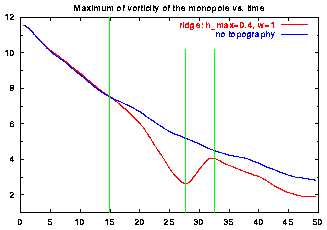
As the monopole moves to the north on the east side of the ridge it is deformed (see above) and partly crosses the top of the ridge. During this time the maximum of vorticity is located to the right of the top. At a certain moment the part of the vortex to the left of the top becomes the strongest one and then the maximum of vorticity is "suddenly" located there. That is the origin of the "jump" in the monopole's trajectory, shown in green in the left graph. This is the moment when the maximum of vorticity reaches a minimal value in the right graph.
For a monopole on a pure beta-plane the value of f0 does not matter. In this case, however, there is a bottom topography present, and a non-zero value has effect on the monopole's evolution. Positive f0-values mean that the ridge is on the northern hemisphere, and for negative f0-values the ridge is on the soutern hemisphere.
For f0-values near f0=0 the effect is not very big, depending on the height of the ridge, but for larger absolute f0-values the effect is considerable. These two example show this clearly:
===> Bessel monopole encounters a north-south
ridge -- with f0=+3.
===> Bessel monopole encounters a north-south
ridge -- with f0=-3.
Contours of potential vorticity, drawn at the same levels as the
contours above, for the case of no topography (left), an east-west ridge
(middle) and a north-south ridge (right).
The latter ridge obviously deforms the potential vorticity from the
pure beta-effect (left) more than the east-west ridge does.
For the pure beta-effect, the contours are straight lines, since
the potential vorticity then equals beta*y (=0.3*y in this case).
The monopole shows up again as a "hole" at (+3,-3).
The evolution of the vorticity distribution is computed with a Finite Difference Method which solves the two-dimensional vorticity (Navier-Stokes) equation. Time and distances are given in dimensionless units.
===> Some details on the computation presented on this page for those who are interested.
<=== Numerical simulations of 2D vortex evolution with a Finite Difference Method.
 Jos van Geffen --
Home |
Site Map |
Contact Me
Jos van Geffen --
Home |
Site Map |
Contact Me