A monopolar vortex, such as the Bessel monopole, will move in the presence of a background rotation, due to the Coriolis force, as explained on the page about a Bessel monopole on a beta-plane. The rotation effects are described in the model with the Coriolis parameter f=f0+beta*y. The value of f0 does not matter for that simulation since there is no bottom topography example. If there is a bottom topography, then things can be quite different.

In the simulations presented here the monopole encounters a circular mountain, as it travels to the north-west due to the beta-effect.

Simulation

Contours of relative vorticity of the initial situation.
The location of the circular mountain is shown by the shaded region.
(You can click on the pictures for a larger version.)

For all graphs: positive contours (0.1 to 2.0 at an interval of 0.1) are drawn solid, negative contours (-0.1 to -2.0 at an interval of -0.1) are drawn dashed, and the zero contour is dotted. Since the maximum of vorticity at the centre of the monopole is much larger than 2.0, the vortex looks like a "hole".
Note that the domain measures 20 by 20 length units -- the graphs show only the central part of this domain.

Turning on the time evolution means that the monopole moves to the north-west, due to the beta-effect, and it encounters the mountain:

The monopole then starts climbing the mountain. First it goes to the south-west, then to the north, after which it leaves the mountain. In this process the monopole is somewhat deformed to a little elliptic, but it recorvers very well.

The monopole then leaves the mountain and continues its travel to the north-west.

This MPEG movie (0.5 Mb; 101 frames) featuring the evolution from T=0 until T=50, shows far more clearly the effect of the mountain on the monopole.

The monopole's motion across the mountain and its maximum of vorticity are shown in these graphs:

Motion of a tracer (red curve) initially placed at the maximum of vorticity of the monopole (where it remains all the time) when the monopole encounters the mountain. Dots are placed along the curve at every 2.5 time units. Note that the scales on both axes differ -- the mountain is circular! The blue line is the monopole's path due to the beta-effect only.

 

Maximum of vorticity versus time of the monopole crossing the mountain (red) and in the case of no topography (blue). The maximum of vorticity reaches the foot of the mountain at T=12.5 and has definitively left it at about T=31.

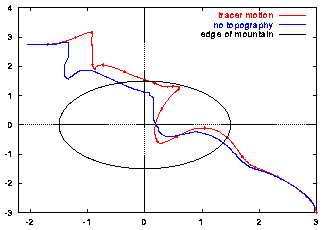
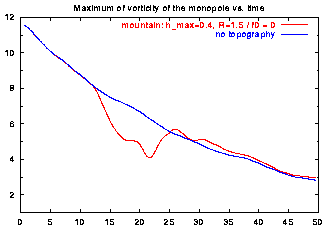
The monopole's rotation about its centre and its motion through the domain create a flow across the topography. This does not mean, however, that relative vorticity is created at the mountain -- as is the case for a uniform flow across a mountain -- since f0=0 in this case.

As a result, the mountain does not have much effect on the monopole's evolution. This is no longer true if the constant f0 in the Coriolis parameter is non-zero.

A value f0=0 means that the mountain is located at the equatior (y=0). For f0 less than zero the mountain is at the southern hemisfere and for f0 larger than zero it is at the northern hemisphere -- which makes quite a difference. The following examples show a very different flow evolution:

===> Bessel monopole encounters a circular mountain -- with f0=-3.
===> Bessel monopole encounters a circular mountain -- with f0=+3.

For details on simulations with different f0-values and heights, see:

Van Geffen, J.H.G.M. and Davies P.A.: 1999,
"A monopolar vortex encounters an isolated topographic feature on a beta-plane,"
Dynam. Atmosph. & Oceans, in press.

The evolution of the vorticity distribution is computed with a Finite Difference Method which solves the two-dimensional vorticity (Navier-Stokes) equation. Time and distances are given in dimensionless units.

===> Some details on the computation presented on this page for those who are interested.

<=== Numerical simulations of 2D vortex evolution with a Finite Difference Method.

 
Jos van Geffen -- Home  |  Site Map  |  Contact Me

created: 21 April 1998
last modified: 26 May 2001