An indication of such an internal clock is given by what Dicke (1978, 1988) calls "the great solar anomaly". Starting with the sunspot maximum in 1761, there are three successive short periods (8.2, 8.7 and 9.7 yr) which add up to 26.6 yr instead of the expected 3 x 11 yr. These three periods are followed by a 17.1-yr period, that makes up for the gain in phase, since the total then is 43.7 yr, close to the expected 4 x 11 yr. According to Dicke (1978) the solar cycle is since 1705 locked to an internal clock; he finds indications that this clock persisted also before 1705, during the Maunder Minimum. The period of this internal oscillator is rather well tuned: 22.422 +/- 0.036 yr (Dicke, 1979b). The phase variations of the sunspot cycle may be caused by fluctuations in the convective transport of the magnetic field from the interior to the surface, with a suggested typical rise time of about 12 yr (Dicke, 1979b, 1988). Gough (1987), however, found "no conclusive evidence for the clock" in the same data set; if anything, the data rather indicate a dynamo model with a finite phase memory. Whitehouse (1985) compared the real sunspot data with a randomly generated data set and finds that phase variations are not random, and he concludes that the "clock model" and the "dynamo model" are both too simple.
Clearly, the available set of data of 25 cycles in the sunspot record is too short for firm conclusions. This is confirmed by a numerical experiment of Barnes et al. (1980) who used a very simple model for simulating the sunspot cycle: a Gaussian noise profile in a narrow band around the cycle period plus a random (white) noise. This model results in a cycle diagram that looks very familiar to the sunspot-record of Fig. 1 and it includes long periods of almost no sunspots; see Fig. 3.
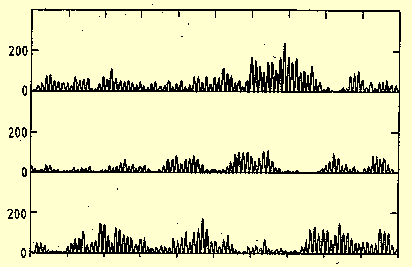Figure 3
Simulation over a period of 3000 years
of annually averaged sunspot numbers.
Each horizontal line is 1000 years long;
the vertical separation of the lines is 400 sunspot numbers.
Compare this graph with the observations shown in
Fig. 1.
[After Barnes et al. (1980).]
The necessity for longer data sets has prompted searches for terrestrial effects of the solar activity cycle. Solar luminosity, i.e. the total energy output, varies along an 11-yr cycle with about 0.1% (Spiegel and Weiss, 1980; Willson and Hudson, 1991). Such small variations result in only small, presumably unresolvable temperature variations on Earth, but they do complicate studies of the greenhouse effect due to human influences. Larger variations in the solar luminosity in the past have had noticeable effects on the Earth's average surface temperature. Lean et al. (1992) estimated, for instance, that during the Maunder Minimum -- a period also known as the "little ice age" -- the solar radiative output was 0.24% below the mean value of 1980-86, resulting in a decrease in the global temperature of 0.2 - 0.6 degrees C.
Another indicator for variations in solar activity is the relative concentration of C-14, which appears to be modulated by the charged particle stream originating from the Sun: a larger solar activity means less C-14, and vice versa. Records of C-14 can be found in tree-rings, in polar ice sheets and in sediments. Other sources of information are the concentration of Be-10 in polar ice and the geomagnetic activity recorded in sediments
Combination of various historic sources has led to a record of long-term changes in solar activity. Short-term changes due to the 11-yr sunspot cycle do not show up in all of the natural records because of the time resolution (for instance due to the lag of time between creation of C-14 and the moment it is absorbed by trees), but in Be-10-records the 11-yr cycle is visible (Beer et al., 1990). A number of authors used some of the above mentioned indicators to find changes in solar activity in the past; see e.g. Eddy (1976, 1977a,b), Dicke (1978, 1979a,b), Stuiver and Quay (1980), Gough (1987) and Gorney (1990). Not only the Maunder Minimum is recovered, but other "grand minima" in solar activity are found (such as the Spörer Minimum, 1415 - 1535), as well as "grand maxima"; see Fig. 4. Eddy (1977a,b) presented 18 periods of major excursions in solar activity over the past 7400 years; there does not seem to be a periodic behaviour in these extrema.
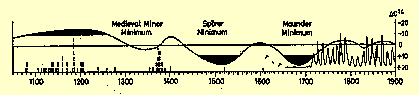Figure 4
Smoothed history of the deviations in the relative atmospheric
C-14 concentration (in parts per million) since the Middle Ages,
where increases of C-14 indicate reduced solar activity.
The thin, peaked curve on the right
represents the mean sunspot record of
Fig. 1;
the vertical bars before 1600 are naked-eye sunspot observations.
[From Clark and Stephenson (1978); see also Eddy (1976).]
There is a correlation between the maximum sunspot numbers and the cycle length which was already mentioned by Wolf in 1861: longer cycles tend to be weaker. This relationship has also been found in some climate indices (e.g. Friis-Christensen and Lassen, 1991; Gribbin, 1991) and in some model calculations (e.g. Stix, 1972; Layzer et al., 1979).
Various periodicities in the range 10 - 400 days have been reported on the basis of several activity indicators (such as X-rays, 10.7 cm radio flux, 10,830 Å helium line, and flare activity; see e.g. Hudson, 1987; Lean and Brueckner, 1989; Silverman, 1990; Pap et al., 1990; Bai and Sturrock, 1991; Carbonell and Ballester, 1992) although not all of these periodicities show up in all of the indicators, so that they do not confirm each other. Moreover, it is unclear whether these periodicities are a permanent feature of solar activity.
Most promising in the field of short periods is helioseismology, which has shown the existence of many acoustic oscillations (p-modes) with a period of about 5 min. At least several hundred thousand globally coherent pressure modes are observable. Most of these p-modes originate presumably beneath the photosphere in the upper layer of the convection zone (for reviews see Deubner and Gough, 1984; Libbrecht, 1988a; Gough and Toomre, 1991). The frequencies of the oscillations vary by a factor of about 0.0001 along the solar cycle (Elsworth et al., 1990; Libbrecht and Woodard, 1990). There have been reports on gravity (g-)modes with a period of 160 min (which equals one-ninth of a day), but this is now believed to be an artifact (Elsworth et al., 1989). In fact, no g-mode has been reliably detected yet.
An interesting investigation on short periods in solar activity has been carried out by Stenflo and Vogel (1986; see also Stenflo, 1991) and Stenflo and Weisenhorn (1987) who used 25 years of magnetograph data and analyzed the radial component of the magnetic field in terms of spherical harmonics of degree l and order m, assuming axisymmetry (i.e. symmetry with respect to the rotation axis, which means that m=0). Even l modes are symmetric and odd l modes are anti-symmetric with respect to the equator, so that l=1 corresponds to the dipole, l=2 to the quadrupole, etc. The major result of Stenflo and co-workers is a clear decoupling between the even and odd l modes for l<=14: only in the odd l modes the 22-yr period is seen in the power spectrum and virtually no higher modes, whereas the even l modes show higher frequencies up to 1.5 yr at l=14. See also Gokhale et al. (1992), who used a longer set of sunspot data and the modes l<=36. The decoupling between the even and odd l modes is consistent with Hale's polarity laws, mentioned at the beginning of this section: the toroidal field, responsible of the magnetic structures in active regions, is purely anti-symmetric with respect to the equator, i.e. has odd parity (odd l).
Forward to 3. The solar dynamo
Back to the contents
Back to the thesis page
Jos van Geffen --
Home |
Site Map |
Contact Me
